Knowledge of the time needed for solidification is crucial for any casting procedure. The rate at which growth of a solid occurs depends on the cooling rate, or the rate of heat extraction. A higher cooling rate produces rapid solidification, or short solidification times. A generic relationship between the solidification time, the volume, and the surface area of a casting can be obtained by dint of Chvorinov’s rule:
In this expression, V is the casting volume, A is the casting surface area, n is an exponent generally taken as 2, and B is a coefficient known as the mold constant. The value of B depends on a series of factors, including the thermophysical properties of the metal and mold material, latent heat of the metal, pouring temperature, and mold geometry. Its value for a given casting operation can be based on experimental data from previous operations carried out using the same mold material, metal, and pouring temperature, even though the shape of the part may be quite different. A quick dimensional analysis reveals that, when n is equal to 2, B has units of time/length², usually min/cm² or min/in.². Chvorinov’s rule basically accounts for the geometry of a casting and the heat transfer conditions, implying, in practical terms, that a casting with a small volume and relatively large surface area will cool more rapidly.
Having introduced Chvorinov’s rule, we now present two practical examples.
Example 1
In casting experiments performed using a certain alloy and type of sand mold, it took 160 seconds for a cube-shaped casting to solidify completely. The cube had a side of 50 mm.
A) Find the mold constant in min/cm².
B) If the same alloy and mold type were used, find the total solidification time for a cylindrical casting of diameter = 30 mm and length = 50 mm.
This is one of the two problem types one may encounter when dealing with Chvorinov’s rule, namely (1) given the casting dimensions and the mold constant, calculate the solidification time; or (2) given the casting time and the casting dimensions, calculate the mold constant. Part A is an example of the latter.
The casting volume and area are V = 5.0³ = 125 cm³ and A = 6 
Having obtained the mold constant, we can determine the casting time for the cylindrical casting proposed in part B – or, for that matter, any casting geometry, provided the alloy and mold type are the same. In other words, we now have a problem of type (1).
The volume of the cylindrical casting is
while the surface area is
Lastly, the solidification time is
Example 2
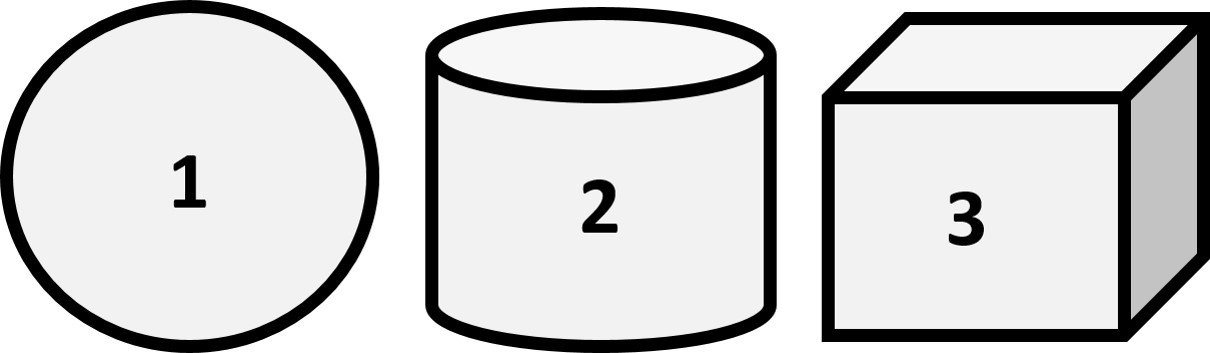
Total solidification times of three geometries are to be compared: (1) a sphere with diameter = 10 cm; (2) a cylinder with diameter and length both = 10 cm; and (3) a cube with each side = 10 cm. The same casting alloy and solidification conditions are used in all three cases. Which casting would solidify faster?
The volume and surface areas of each part are listed below.
We don’t have the mold constant B, but we were told that the alloy used and conditions are the same for the three parts. Accordingly, B is the same for all three cases. The solidification time for the sphere is
The solidification time for the cylinder, in turn, is
Lastly, the solidification time for the cube is
Surprisingly,
Go further
Montogue offers a full quiz on casting and flow stress, which you can find here. Students of metallurgical engineering may also enjoy our materials on bulk deformation processes and heat treatment of steel. Check them out!
References
• ASKELAND, D., FULAY, P., and WRIGHT, W. (2011). The Science and Engineering of Materials. 6th edition. Boston: Cengage Learning.
• GROOVER, M. (2013). Fundamentals of Modern Manufacturing. 5th edition. Hoboken: John Wiley and Sons.
